Posts tagged as “dp”

花花酱 LeetCode 664. Strange Printer

By zxi on September 20, 2017

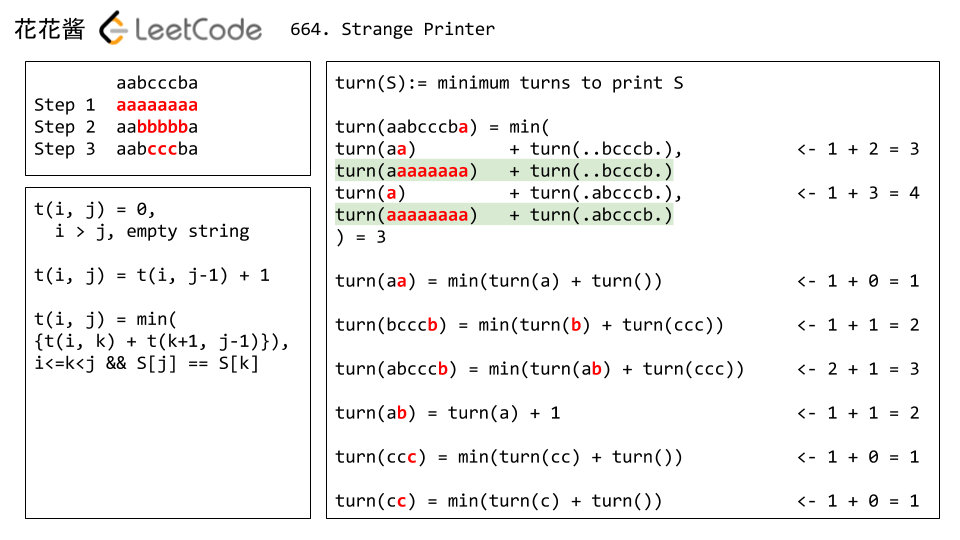
Problem:

There is a strange printer with the following two special requirements:

The printer can only print a sequence of the same character each time.
At each turn, the printer can print new characters starting from and ending at any places, and will cover the original existing characters.

Given a string consists of lower English letters only, your job is to count the minimum number of turns the printer needed in order to print it.

Example 1:

Input: "aaabbb"

Output: 2

Explanation: Print "aaa" first and then print "bbb".

Example 2:

Input: "aba"

Output: 2

Explanation: Print "aaa" first and then print "b" from the second place of the string, which will cover the existing character 'a'.

Hint: Length of the given string will not exceed 100.

Idea:

Dynamic programming

Time Complexity:

O(n^3)

Space Complexity:

O(n^2)

Solution:

C++

// Author: Huahua

// Time Complexity: O(n^3)

// Space Complexity: O(n^2)

// Running Time: 22 ms

class Solution {

public:

int strangePrinter(const string& s) {

int l = s.length();

t_ = vector<vector<int>>(l, vector<int>(l, 0));

return turn(s, 0, s.length() - 1);

}

private:

// Minimal turns to print s[i] to s[j]

int turn(const string& s, int i, int j) {

// Empty string

if (i > j) return 0;

// Solved

if (t_[i][j] > 0) return t_[i][j];

// Default behavior, print s[i] to s[j-1] and print s[j]

int ans = turn(s, i, j-1) + 1;

for (int k = i; k < j; ++k)

if (s[k] == s[j])

ans = min(ans, turn(s, i, k) + turn(s, k + 1, j - 1));

return t_[i][j] = ans;

}

vector<vector<int>> t_;

};

Java

// Author: Huahua

// Time Complexity: O(n^3)

// Space Complexity: O(n^2)

// Running Time: 31 ms (beat 99.25%) 9/2017

class Solution {

public int strangePrinter(String s) {

int l = s.length();

t_ = new int[l][l];

return turn(s.toCharArray(), 0, l - 1);

}

// Minimal turns to print s[i] to s[j]

private int turn(char[] s, int i, int j) {

// Empty string

if (i > j) return 0;

// Solved

if (t_[i][j] > 0) return t_[i][j];

// Default behavior, print s[i] to s[j-1] and print s[j]

int ans = turn(s, i, j-1) + 1;

for (int k = i; k < j; ++k)

if (s[k] == s[j])

ans = Math.min(ans, turn(s, i, k) + turn(s, k + 1, j - 1));

return t_[i][j] = ans;

}

private int[][] t_;

}

Python3

"""

Author: Huahua

Time Complexity: O(n^3)

Space Complexity: O(n^2)

Running Time: 895 ms (beat 66%) 9/2017

"""

class Solution(object):

def strangePrinter(self, s):

"""

:type s: str

:rtype: int

"""

l = len(s)

self._t = [[0 for _ in xrange(l)] for _ in xrange(l)]

return self._turns(s, 0, l - 1)

def _turns(self, s, i, j):

if i > j: return 0

if self._t[i][j] > 0: return self._t[i][j]

ans = self._turns(s, i, j - 1) + 1

for k in xrange(i, j):

if s[k] == s[j]:

ans = min(ans, self._turns(s, i, k)

+ self._turns(s, k + 1, j-1))

self._t[i][j] = ans

return self._t[i][j]

花花酱 LeetCode 304. Range Sum Query 2D – Immutable

By zxi on September 18, 2017

https://leetcode.com/problems/range-sum-query-2d-immutable/description/

Problem:

Given a 2D matrix matrix, find the sum of the elements inside the rectangle defined by its upper left corner (row1, col1) and lower right corner (row2, col2).

The above rectangle (with the red border) is defined by (row1, col1) = (2, 1) and (row2, col2) = (4, 3), which contains sum = 8.

Example:

Given matrix = [

[3, 0, 1, 4, 2],

[5, 6, 3, 2, 1],

[1, 2, 0, 1, 5],

[4, 1, 0, 1, 7],

[1, 0, 3, 0, 5]

]

sumRegion(2, 1, 4, 3) : 8

sumRegion(1, 1, 2, 2) : 11

sumRegion(1, 2, 2, 4) : 12

Note:

You may assume that the matrix does not change.
There are many calls to sumRegion function.
You may assume that row1 ≤ row2 and col1 ≤ col2.

Idea:

Dynamic programming

Time complexity:

O(n^2)

sumRegion: O(1)

Solution:

// Author: Huahua

// Time complexity:

// constructor: O(n^2)

// sumRegion: O(1)

// Running time: 22 ms 9/2017

class NumMatrix {

public:

NumMatrix(const vector<vector<int>>& matrix) {

sums_.clear();

if (matrix.empty()) return;

int m = matrix.size();

int n = matrix[0].size();

// sums_[i][j] = sum(matrix[0][0] ~ matrix[i-1][j-1])

sums_ = vector<vector<int>>(m + 1, vector<int>(n + 1, 0));

for (int i = 1; i <= m; ++i)

for (int j = 1; j <= n; ++j)

sums_[i][j] = matrix[i - 1][j - 1]

+ sums_[i - 1][j]

+ sums_[i][j - 1]

- sums_[i - 1][j - 1];

}

int sumRegion(int row1, int col1, int row2, int col2) {

return sums_[row2 + 1][col2 + 1]

- sums_[row2 + 1][col1]

- sums_[row1][col2 + 1]

+ sums_[row1][col1];

}

private:

vector<vector<int>> sums_;

};

/**

* Your NumMatrix object will be instantiated and called as such:

* NumMatrix obj = new NumMatrix(matrix);

* int param_1 = obj.sumRegion(row1,col1,row2,col2);

花花酱 LeetCode 221. Maximal Square

By zxi on September 18, 2017

Problem:

Given a 2D binary matrix filled with 0’s and 1’s, find the largest square containing only 1’s and return its area.

For example, given the following matrix:

1 0 1 0 0
1 0 1 1 1
1 1 1 1 1
1 0 0 1 0

Return 4.

Idea:

Dynamic programming

Solution 0: O(n^5)

Solution 1: O(n^3)

Solution 2: O(n^2)

Solution 1:

// Author: Huahua

// Time complexity: O(n^3)

// Running time: 39 ms

class Solution {

public:

int maximalSquare(vector<vector<char>>& matrix) {

if (matrix.empty()) return 0;

int m = matrix.size();

int n = matrix[0].size();

// sums[i][j] = sum(matrix[0][0] ~ matrix[i-1][j-1])

vector<vector<int>> sums(m + 1, vector<int>(n + 1, 0));

for (int i = 1; i <= m; ++i)

for (int j = 1; j <= n; ++j)

sums[i][j] = matrix[i - 1][j - 1] - '0'

+ sums[i - 1][j]

+ sums[i][j - 1]

- sums[i - 1][j - 1];

int ans = 0;

for (int i = 1; i <= m; ++i)

for (int j = 1; j <= n; ++j)

for (int k = min(m - i + 1, n - j + 1); k > 0; --k) {

int sum = sums[i + k - 1][j + k - 1]

- sums[i + k - 1][j - 1]

- sums[i - 1][j + k - 1]

+ sums[i - 1][j - 1];

// full of 1

if (sum == k*k) {

ans = max(ans, sum);

break;

}

return ans;

}

};

Solution 2:

// Author: Huahua

// Time complexity: O(n^2)

// Running time: 6 ms

class Solution {

public:

int maximalSquare(vector<vector<char>>& matrix) {

if (matrix.empty()) return 0;

int m = matrix.size();

int n = matrix[0].size();

vector<vector<int>> sizes(m, vector<int>(n, 0));

int ans = 0;

for (int i = 0; i < m; ++i)

for (int j = 0; j < n; ++j) {

sizes[i][j] = matrix[i][j] - '0';

if (!sizes[i][j]) continue;

if (i == 0 || j == 0) {

// do nothing

} else if (i == 0)

sizes[i][j] = sizes[i][j - 1] + 1;

else if (j == 0)

sizes[i][j] = sizes[i - 1][j] + 1;

else

sizes[i][j] = min(min(sizes[i - 1][j - 1],

sizes[i - 1][j]),

sizes[i][j - 1]) + 1;

ans = max(ans, sizes[i][j]*sizes[i][j]);

}

return ans;

}

};

花花酱 LeetCode 673. Number of Longest Increasing Subsequence

By zxi on September 15, 2017

https://leetcode.com/problems/number-of-longest-increasing-subsequence

Problem:

Given an unsorted array of integers, find the number of longest increasing subsequence.

Example 1:

Input: [1,3,5,4,7]

Output: 2

Explanation: The two longest increasing subsequence are [1, 3, 4, 7] and [1, 3, 5, 7].

Example 2:

Input: [2,2,2,2,2]

Output: 5

Explanation: The length of longest continuous increasing subsequence is 1, and there are 5 subsequences' length is 1, so output 5.

Note: Length of the given array will be not exceed 2000 and the answer is guaranteed to be fit in 32-bit signed int.

Idea:

Dynamic programming

Solution1:

// Author: Huahua

// Running time: 82 ms

class Solution {

public:

int findNumberOfLIS(vector<int>& nums) {

int n = nums.size();

if (n == 0) return 0;

c_ = vector<int>(n, 0);

l_ = vector<int>(n, 0);

// Find the length LIS.

int max_len = 0;

for (int i = 0; i < n; ++i)

max_len = max(max_len, len(nums, i));

// Checking all endings.

int ans = 0;

for (int i = 0; i < n; ++i)

if (len(nums, i) == max_len)

ans += count(nums, i);

return ans;

}

private:

vector<int> c_; // c[i]: number of LIS ends with nums[i] / NLIS'

vector<int> l_; // l[i]: lengeh of LIS ends with nums[i] / LIS'

// Number of LIS ends with nums[n]

int count(const vector<int>& nums, int n) {

if (n == 0) return 1;

if (c_[n] > 0) return c_[n];

int total_count = 0;

int l = len(nums, n);

for (int i = 0; i < n; ++i)

if (nums[n] > nums[i] && len(nums, i) == l-1)

total_count += count(nums, i);

if (total_count == 0)

total_count = 1;

return c_[n] = total_count;

}

// Length of LIS ends with nums[n]

int len(const vector<int>& nums, int n) {

if (n == 0) return 1;

if (l_[n] > 0) return l_[n];

int max_len = 1;

// Check every subarray

for (int i = 0; i < n; ++i)

if (nums[n] > nums[i])

max_len = max(max_len, len(nums, i) + 1);

return l_[n] = max_len;

}

};

Solution2:

// Author: Huahua

// Running time: 33 ms

class Solution {

public:

int findNumberOfLIS(vector<int>& nums) {

int n = nums.size();

if (n == 0) return 0;

vector<int> c(n, 1);

vector<int> l(n, 1);

for (int i = 1; i < n; ++i)

for(int j = 0; j < i; ++j)

if (nums[i] > nums[j]) {

if (l[j] + 1 > l[i]) {

l[i] = l[j] + 1;

c[i] = c[j];

} else if (l[j] + 1 == l[i]) {

c[i] += c[j];

}

int max_len = *max_element(l.begin(), l.end());

int ans = 0;

for (int i = 0; i < n; ++i)

if (l[i] == max_len)

ans += c[i];

return ans;

}

};

Related Problems:

花花酱 LeetCode 300. Longest Increasing Subsequence

By zxi on September 10, 2017

Problem:

Given an unsorted array of integers, find the length of longest increasing subsequence.

For example,
Given [10, 9, 2, 5, 3, 7, 101, 18],
The longest increasing subsequence is [2, 3, 7, 101], therefore the length is 4. Note that there may be more than one LIS combination, it is only necessary for you to return the length.

Your algorithm should run in O(n²) complexity.

Follow up: Could you improve it to O(n log n) time complexity?

Idea:

Dynamic Programming

Time Complexity:

O(n^2)

Solution 1:

// Author: Huahua

// Running time: 29 ms

class Solution {

public:

int lengthOfLIS(vector<int>& nums) {

if (nums.empty()) return 0;

int n = nums.size();

auto f = vector<int>(n, 1);

for (int i = 1; i < n; ++i)

for (int j = 0; j < i; ++j)

if (nums[i] > nums[j])

f[i] = max(f[i], f[j] + 1);

return *max_element(f.begin(), f.end());

}

};

Solution 2: DP + Binary Search / Patience Sort

dp[i] := smallest tailing number of a increasing subsequence of length i + 1.

dp is an increasing array, we can use binary search to find the index to insert/update the array.

ans = len(dp)

Time complexity: O(nlogn)
Space complexity: O(n)

C++

class Solution {

public:

int lengthOfLIS(vector<int>& nums) {

const int n = nums.size();

if (n == 0) return 0;

vector<int> dp;

for (int i = 0; i < n; ++i) {

auto it = lower_bound(begin(dp), end(dp), nums[i]);

if (it == end(dp))

dp.push_back(nums[i]);

else

*it = nums[i];

}

return dp.size();

}

};

Python3

class Solution:

def lengthOfLIS(self, nums: List[int]) -> int:

d = []

for x in nums:

i = bisect_left(d, x)

if i == len(d):

d.append(x)

else:

d[i] = x

return len(d)

Related Problems: