Posts tagged as “recursion”

花花酱 LeetCode 2265. Count Nodes Equal to Average of Subtree

By zxi on May 10, 2022

Given the root of a binary tree, return the number of nodes where the value of the node is equal to the average of the values in its subtree.

Note:

The average of n elements is the sum of the n elements divided by n and rounded down to the nearest integer.
A subtree of root is a tree consisting of root and all of its descendants.

Example 1:

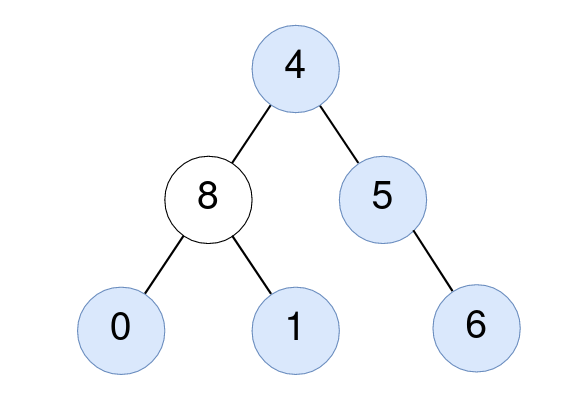
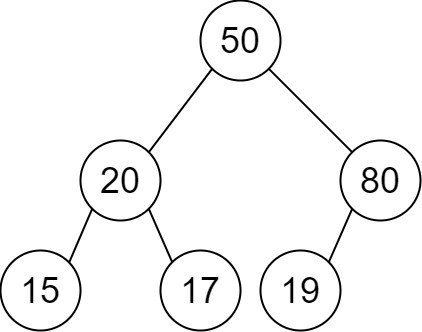
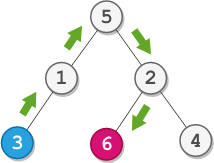
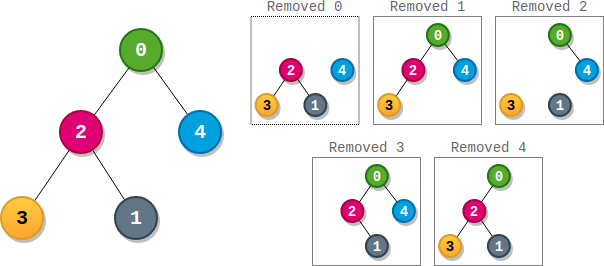
Input: root = [4,8,5,0,1,null,6]
Output: 5
Explanation: 
For the node with value 4: The average of its subtree is (4 + 8 + 5 + 0 + 1 + 6) / 6 = 24 / 6 = 4.
For the node with value 5: The average of its subtree is (5 + 6) / 2 = 11 / 2 = 5.
For the node with value 0: The average of its subtree is 0 / 1 = 0.
For the node with value 1: The average of its subtree is 1 / 1 = 1.
For the node with value 6: The average of its subtree is 6 / 1 = 6.

Example 2:

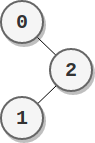
Input: root = [1]
Output: 1
Explanation: For the node with value 1: The average of its subtree is 1 / 1 = 1.

Constraints:

The number of nodes in the tree is in the range [1, 1000].
0 <= Node.val <= 1000

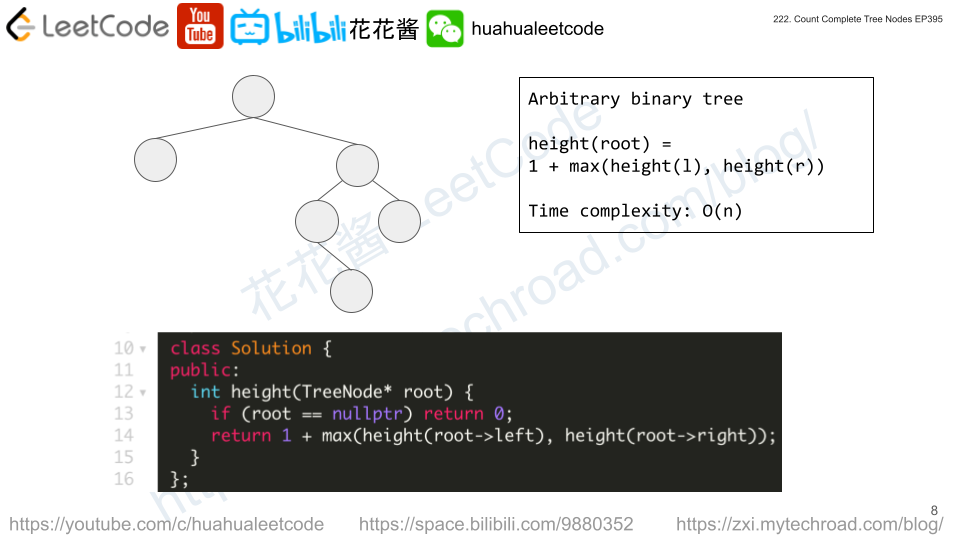
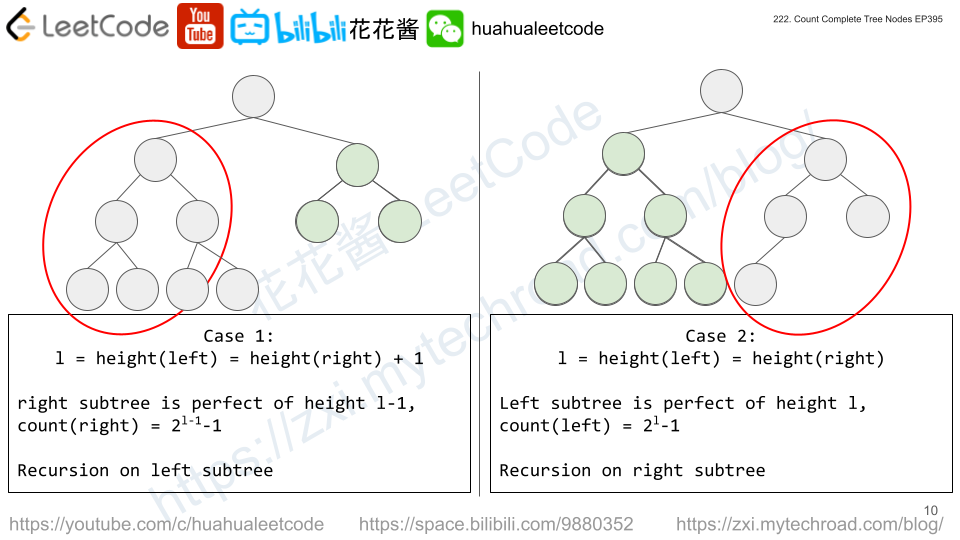
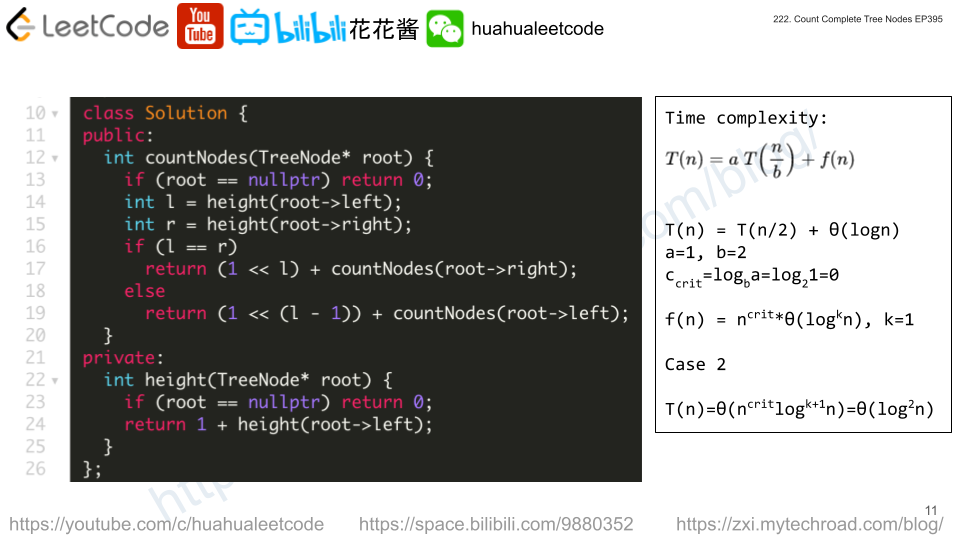
Solution: Recursion

Time complexity: O(n)
Space complexity: O(h)

C++

// Author: Huahua

class Solution {

public:

int averageOfSubtree(TreeNode* root) {

// Returns {sum(val), sum(node), ans}

function<tuple<int, int, int>(TreeNode*)> getSum

= [&](TreeNode* root) -> tuple<int, int, int> {

if (!root) return {0, 0, 0};

auto [lv, lc, la] = getSum(root->left);

auto [rv, rc, ra] = getSum(root->right);

int sum = lv + rv + root->val;

int count = lc + rc + 1;

int ans = la + ra + (root->val == sum / count);

return {sum, count, ans};

};

return get<2>(getSum(root));

}

};

花花酱 LeetCode 2196. Create Binary Tree From Descriptions

By zxi on March 8, 2022

You are given a 2D integer array descriptions where descriptions[i] = [parent_i, child_i, isLeft_i] indicates that parent_i is the parent of child_i in a binary tree of unique values. Furthermore,

If isLeft_i == 1, then child_i is the left child of parent_i.
If isLeft_i == 0, then child_i is the right child of parent_i.

Construct the binary tree described by descriptions and return its root.

The test cases will be generated such that the binary tree is valid.

Example 1:

Input: descriptions = [[20,15,1],[20,17,0],[50,20,1],[50,80,0],[80,19,1]]
Output: [50,20,80,15,17,19]
Explanation: The root node is the node with value 50 since it has no parent.
The resulting binary tree is shown in the diagram.

Example 2:

Input: descriptions = [[1,2,1],[2,3,0],[3,4,1]]
Output: [1,2,null,null,3,4]
Explanation: The root node is the node with value 1 since it has no parent.
The resulting binary tree is shown in the diagram.

Constraints:

1 <= descriptions.length <= 10⁴
descriptions[i].length == 3
1 <= parent_i, child_i <= 10⁵
0 <= isLeft_i <= 1
The binary tree described by descriptions is valid.

Solution: Hashtable + Recursion

Use one hashtable to track the children of each node.
Use another hashtable to track the parent of each node.
Find the root who doesn’t have parent.
Build the tree recursively from root.

Time complexity: O(n)
Space complexity: O(n)

C++

// Author: Huahua

class Solution {

public:

TreeNode* createBinaryTree(vector<vector<int>>& descriptions) {

unordered_set<int> hasParent;

unordered_map<int, pair<int, int>> children;

for (const auto& d : descriptions) {

hasParent.insert(d[1]);

(d[2] ? children[d[0]].first : children[d[0]].second) = d[1];

}

int root = -1;

for (const auto& d : descriptions)

if (!hasParent.count(d[0])) root = d[0];

function<TreeNode*(int)> build = [&](int cur) -> TreeNode* {

if (!cur) return nullptr;

return new TreeNode(cur,

build(children[cur].first),

build(children[cur].second));

};

return build(root);

}

};

花花酱 LeetCode 222. Count Complete Tree Nodes

By zxi on December 7, 2021

Given the root of a complete binary tree, return the number of the nodes in the tree.

According to Wikipedia, every level, except possibly the last, is completely filled in a complete binary tree, and all nodes in the last level are as far left as possible. It can have between 1 and 2^h nodes inclusive at the last level h.

Design an algorithm that runs in less than O(n) time complexity.

Example 1:

Input: root = [1,2,3,4,5,6]
Output: 6

Example 2:

Input: root = []
Output: 0

Example 3:

Input: root = [1]
Output: 1

Constraints:

The number of nodes in the tree is in the range [0, 5 * 10⁴].
0 <= Node.val <= 5 * 10⁴
The tree is guaranteed to be complete.

Solution: Recursion

For each node, count the height of it’s left and right subtree by going left only.

Let L = height(left) R = height(root), if L == R, which means the left subtree is perfect.
It has (2^L – 1) nodes, +1 root, we only need to count nodes of right subtree recursively.
If L != R, L must be R + 1 since the tree is complete, which means the right subtree is perfect.
It has (2^(L-1) – 1) nodes, +1 root, we only need to count nodes of left subtree recursively.

Time complexity: T(n) = T(n/2) + O(logn) = O(logn*logn)

Space complexity: O(logn)

C++

// Author: Huahua

class Solution {

public:

int countNodes(TreeNode* root) {

if (root == nullptr) return 0;

int l = depth(root->left);

int r = depth(root->right);

if (l == r)

return (1 << l) + countNodes(root->right);

else

return (1 << (l - 1)) + countNodes(root->left);

}

private:

int depth(TreeNode* root) {

if (root == nullptr) return 0;

return 1 + depth(root->left);

}

};

花花酱 LeetCode 2096. Step-By-Step Directions From a Binary Tree Node to Another

By zxi on December 5, 2021

You are given the root of a binary tree with n nodes. Each node is uniquely assigned a value from 1 to n. You are also given an integer startValue representing the value of the start node s, and a different integer destValue representing the value of the destination node t.

Find the shortest path starting from node s and ending at node t. Generate step-by-step directions of such path as a string consisting of only the uppercase letters 'L', 'R', and 'U'. Each letter indicates a specific direction:

'L' means to go from a node to its left child node.
'R' means to go from a node to its right child node.
'U' means to go from a node to its parent node.

Return the step-by-step directions of the shortest path from node s to node t.

Example 1:

Input: root = [5,1,2,3,null,6,4], startValue = 3, destValue = 6
Output: "UURL"
Explanation: The shortest path is: 3 → 1 → 5 → 2 → 6.

Example 2:

Input: root = [2,1], startValue = 2, destValue = 1
Output: "L"
Explanation: The shortest path is: 2 → 1.

Constraints:

The number of nodes in the tree is n.
2 <= n <= 10⁵
1 <= Node.val <= n
All the values in the tree are unique.
1 <= startValue, destValue <= n
startValue != destValue

Solution: Lowest common ancestor

It’s no hard to see that the shortest path is from the start node to the lowest common ancestor (LCA) of (start, end), then to the end node. The key is to find the LCA while finding paths from root to two nodes.

We can use recursion to find/build a path from root to a target node.
The common prefix of these two paths is the path from root to the LCA that we need to remove from the shortest path.
e.g.
root to start “LLRLR”
root to dest “LLLR”
common prefix is “LL”, after removing, it becomes:
LCA to start “RLR”
LCA to dest “LR”
Final path becomes “UUU” + “LR” = “UUULR”

The final step is to replace the L/R with U for the start path since we are moving up and then concatenate with the target path.

Time complexity: O(n)
Space complexity: O(n)

C++

// Author: Huahua

class Solution {

public:

string getDirections(TreeNode* root, int startValue, int destValue) {

string startPath;

string destPath;

buildPath(root, startValue, startPath);

buildPath(root, destValue, destPath);

// Remove common suffix (shared path from root to LCA)

while (!startPath.empty() && !destPath.empty()

&& startPath.back() == destPath.back()) {

startPath.pop_back();

destPath.pop_back();

}

reverse(begin(destPath), end(destPath));

return string(startPath.size(), 'U') + destPath;

}

private:

bool buildPath(TreeNode* root, int t, string& path) {

if (!root) return false;

if (root->val == t) return true;

if (buildPath(root->left, t, path)) {

path.push_back('L');

return true;

} else if (buildPath(root->right, t, path)) {

path.push_back('R');

return true;

}

return false;

}

};

花花酱 LeetCode 2049. Count Nodes With the Highest Score

By zxi on October 26, 2021

There is a binary tree rooted at 0 consisting of n nodes. The nodes are labeled from 0 to n - 1. You are given a 0-indexed integer array parents representing the tree, where parents[i] is the parent of node i. Since node 0 is the root, parents[0] == -1.

Each node has a score. To find the score of a node, consider if the node and the edges connected to it were removed. The tree would become one or more non-empty subtrees. The size of a subtree is the number of the nodes in it. The score of the node is the product of the sizes of all those subtrees.

Return the number of nodes that have the highest score.

Example 1:

Input: parents = [-1,2,0,2,0]
Output: 3
Explanation:
- The score of node 0 is: 3 * 1 = 3
- The score of node 1 is: 4 = 4
- The score of node 2 is: 1 * 1 * 2 = 2
- The score of node 3 is: 4 = 4
- The score of node 4 is: 4 = 4
The highest score is 4, and three nodes (node 1, node 3, and node 4) have the highest score.

Example 2:

Input: parents = [-1,2,0]
Output: 2
Explanation:
- The score of node 0 is: 2 = 2
- The score of node 1 is: 2 = 2
- The score of node 2 is: 1 * 1 = 1
The highest score is 2, and two nodes (node 0 and node 1) have the highest score.

Constraints:

n == parents.length
2 <= n <= 10⁵
parents[0] == -1
0 <= parents[i] <= n - 1 for i != 0
parents represents a valid binary tree.

Solution: Recursion

Write a function that returns the element of a subtree rooted at node.

We can compute the score based on:
1. size of the subtree(s)
2. # of children

Root is a special case whose score is max(c[0], 1) * max(c[1], 1).

Time complexity: O(n)
Space complexity: O(n)

C++

// Author: Huahua

class Solution {

public:

int countHighestScoreNodes(vector<int>& parents) {

const int n = parents.size();

long high = 0;

int ans = 0;

vector<vector<int>> tree(n);

for (int i = 1; i < n; ++i)

tree[parents[i]].push_back(i);

function<int(int)> dfs = [&](int node) -> int {

long c[2] = {0, 0};

for (int i = 0; i < tree[node].size(); ++i)

c[i] = dfs(tree[node][i]);

long score = 0;

if (node == 0) // case #1: root

score = max(c[0], 1l) * max(c[1], 1l);

else if (tree[node].size() == 0) // case #2: leaf

score = n - 1;

else if (tree[node].size() == 1) // case #3: one child

score = c[0] * (n - c[0] - 1);

else // case #4: two children

score = c[0] * c[1] * (n - c[0] - c[1] - 1);

if (score > high) {

high = score;

ans = 1;

} else if (score == high) {

++ans;

}

return 1 + c[0] + c[1];

};

dfs(0);

return ans;

}

};