花花酱 LeetCode 2476. Closest Nodes Queries in a Binary Search Tree

You are given the root of a binary search tree and an array queries of size n consisting of positive integers.

Find a 2D array answer of size n where answer[i] = [mini, maxi]:

• mini is the largest value in the tree that is smaller than or equal to queries[i]. If a such value does not exist, add -1 instead.
• maxi is the smallest value in the tree that is greater than or equal to queries[i]. If a such value does not exist, add -1 instead.

Return the array answer.

Example 1:

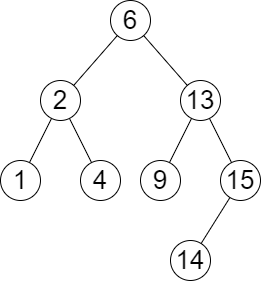
Input: root = [6,2,13,1,4,9,15,null,null,null,null,null,null,14], queries = [2,5,16]
Output: [[2,2],[4,6],[15,-1]]
Explanation: We answer the queries in the following way:
- The largest number that is smaller or equal than 2 in the tree is 2, and the smallest number that is greater or equal than 2 is still 2. So the answer for the first query is [2,2].
- The largest number that is smaller or equal than 5 in the tree is 4, and the smallest number that is greater or equal than 5 is 6. So the answer for the second query is [4,6].
- The largest number that is smaller or equal than 16 in the tree is 15, and the smallest number that is greater or equal than 16 does not exist. So the answer for the third query is [15,-1].

Example 2:

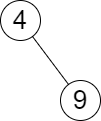
Input: root = [4,null,9], queries = [3]
Output: [[-1,4]]
Explanation: The largest number that is smaller or equal to 3 in the tree does not exist, and the smallest number that is greater or equal to 3 is 4. So the answer for the query is [-1,4].

Constraints:

• The number of nodes in the tree is in the range [2, 105].
• 1 <= Node.val <= 106
• n == queries.length
• 1 <= n <= 105
• 1 <= queries[i] <= 106

Solution: Convert to sorted array

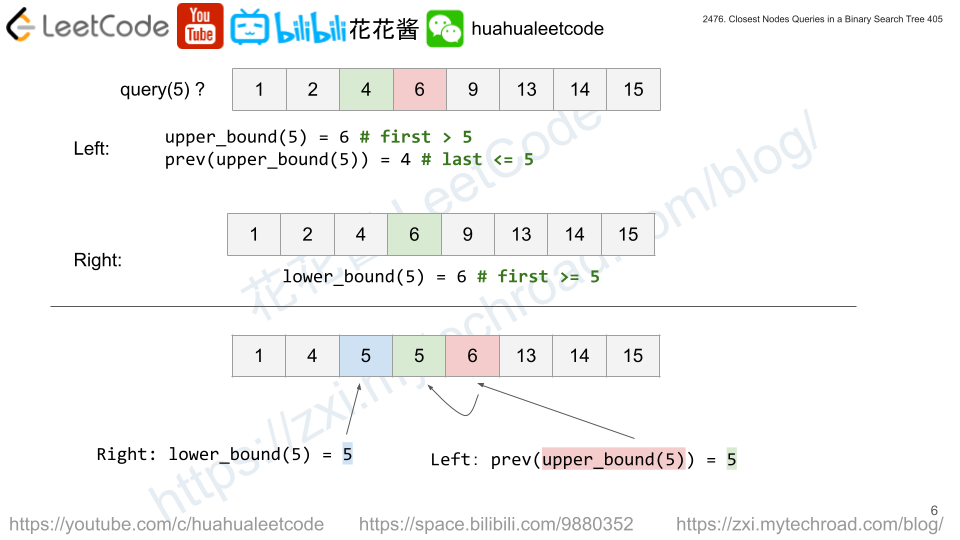
Since we don’t know whether the tree is balanced or not, the safest and easiest way is to convert the tree into a sorted array using inorder traversal. Or just any traversal and sort the array later on.

Once we have a sorted array, we can use lower_bound / upper_bound to query.

Time complexity: O(qlogn)
Space complexity: O(n)

One binary search per query.