Posts tagged as “treeset”

花花酱 LeetCode 2007. Find Original Array From Doubled Array

By zxi on November 25, 2021

An integer array original is transformed into a doubled array changed by appending twice the value of every element in original, and then randomly shuffling the resulting array.

Given an array changed, return original if changed is a doubled array. If changed is not a doubled array, return an empty array. The elements in original may be returned in any order.

Example 1:

Input: changed = [1,3,4,2,6,8]
Output: [1,3,4]
Explanation: One possible original array could be [1,3,4]:
- Twice the value of 1 is 1 * 2 = 2.
- Twice the value of 3 is 3 * 2 = 6.
- Twice the value of 4 is 4 * 2 = 8.
Other original arrays could be [4,3,1] or [3,1,4].

Example 2:

Input: changed = [6,3,0,1]
Output: []
Explanation: changed is not a doubled array.

Example 3:

Input: changed = [1]
Output: []
Explanation: changed is not a doubled array.

Constraints:

1 <= changed.length <= 10⁵
0 <= changed[i] <= 10⁵

Solution 1: Multiset

Start from the smallest number x, erase one x * 2 from the set.

Time complexity: O(nlogn)
Space complexity: O(n)

C++/Multiset

// Author: Huahua

class Solution {

public:

vector<int> findOriginalArray(vector<int>& changed) {

if (changed.size() & 1) return {};

const int n = changed.size() / 2;

multiset<int> s(begin(changed), end(changed));

while (ans.size() != n) {

int o = *begin(s);

s.erase(begin(s));

ans.push_back(o);

auto it = s.find(o * 2);

if (it == end(s)) return {};

s.erase(it);

}

return ans;

}

};

Solution 2: Hashtable

Time complexity: O(max(nums) + n)
Space complexity: O(max(nums))

C++/Hashtable

// Author: Huahua

class Solution {

public:

vector<int> findOriginalArray(vector<int>& changed) {

if (changed.size() & 1) return {};

const int n = changed.size() / 2;

const int kMax = *max_element(begin(changed), end(changed));

vector<int> m(kMax + 1);

for (int x : changed) ++m[x];

if (m[0] & 1) return {};

vector<int> ans(m[0] / 2, 0);

for (int x = 1; ans.size() != n; ++x) {

if (x * 2 > kMax || m[x * 2] < m[x]) return {};

ans.insert(end(ans), m[x], x);

m[x * 2] -= m[x];

}

return ans;

}

};

花花酱 LeetCode 1845. Seat Reservation Manager

By zxi on May 1, 2021

Design a system that manages the reservation state of n seats that are numbered from 1 to n.

Implement the SeatManager class:

SeatManager(int n) Initializes a SeatManager object that will manage n seats numbered from 1 to n. All seats are initially available.
int reserve() Fetches the smallest-numbered unreserved seat, reserves it, and returns its number.
void unreserve(int seatNumber) Unreserves the seat with the given seatNumber.

Example 1:

Input
["SeatManager", "reserve", "reserve", "unreserve", "reserve", "reserve", "reserve", "reserve", "unreserve"]
[[5], [], [], [2], [], [], [], [], [5]]
Output

[null, 1, 2, null, 2, 3, 4, 5, null]

Explanation SeatManager seatManager = new SeatManager(5); // Initializes a SeatManager with 5 seats. seatManager.reserve(); // All seats are available, so return the lowest numbered seat, which is 1. seatManager.reserve(); // The available seats are [2,3,4,5], so return the lowest of them, which is 2. seatManager.unreserve(2); // Unreserve seat 2, so now the available seats are [2,3,4,5]. seatManager.reserve(); // The available seats are [2,3,4,5], so return the lowest of them, which is 2. seatManager.reserve(); // The available seats are [3,4,5], so return the lowest of them, which is 3. seatManager.reserve(); // The available seats are [4,5], so return the lowest of them, which is 4. seatManager.reserve(); // The only available seat is seat 5, so return 5. seatManager.unreserve(5); // Unreserve seat 5, so now the available seats are [5].

Constraints:

1 <= n <= 10⁵
1 <= seatNumber <= n
For each call to reserve, it is guaranteed that there will be at least one unreserved seat.
For each call to unreserve, it is guaranteed that seatNumber will be reserved.
At most 10⁵ calls in total will be made to reserve and unreserve.

Solution: TreeSet

Time complexity: O(nlogn)
Space complexity: O(n)

C++

// Author: Huahua

class SeatManager {

public:

SeatManager(int n) {

for (int i = 1; i <= n; ++i)

s_.insert(i);

}

int reserve() {

int seat = *begin(s_);

s_.erase(begin(s_));

return seat;

}

void unreserve(int seatNumber) {

s_.insert(seatNumber);

}

private:

set<int> s_;

};

花花酱 LeetCode 1675. Minimize Deviation in Array

By zxi on November 29, 2020

You are given an array nums of n positive integers.

You can perform two types of operations on any element of the array any number of times:

If the element is even, divide it by 2.
- For example, if the array is [1,2,3,4], then you can do this operation on the last element, and the array will be [1,2,3,2].
If the element is odd, multiply it by 2.
- For example, if the array is [1,2,3,4], then you can do this operation on the first element, and the array will be [2,2,3,4].

The deviation of the array is the maximum difference between any two elements in the array.

Return the minimum deviation the array can have after performing some number of operations.

Example 1:

Input: nums = [1,2,3,4]
Output: 1
Explanation: You can transform the array to [1,2,3,2], then to [2,2,3,2], then the deviation will be 3 - 2 = 1.

Example 2:

Input: nums = [4,1,5,20,3]
Output: 3
Explanation: You can transform the array after two operations to [4,2,5,5,3], then the deviation will be 5 - 2 = 3.

Example 3:

Input: nums = [2,10,8]
Output: 3

Constraints:

n == nums.length
2 <= n <= 10⁵
1 <= nums[i] <= 10⁹

Solution: Priority Queue

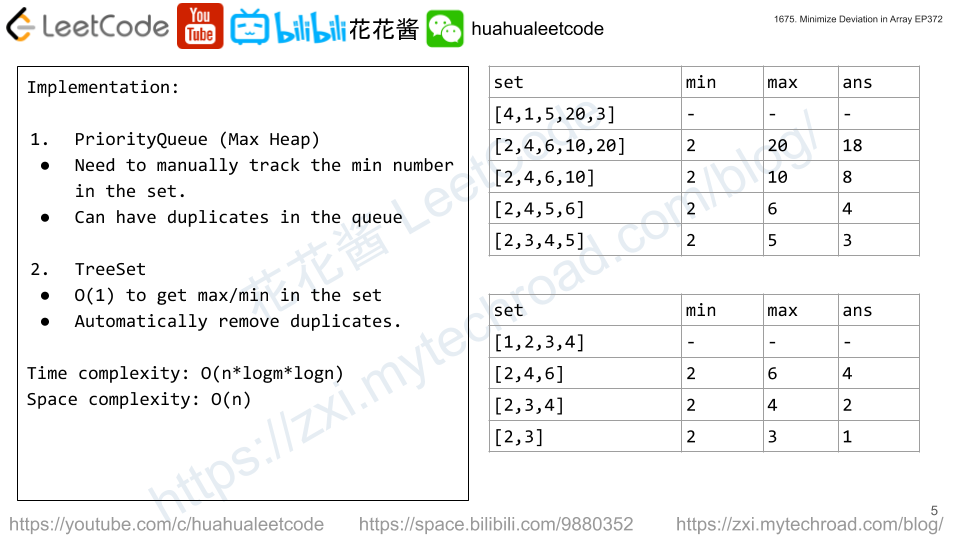
If we double an odd number it becomes an even number, then we can only divide it by two which gives us back the original number. So we can pre-double all the odd numbers and only do division in the following process.

We push all numbers including pre-doubled odd ones onto a priority queue, and track the difference between the largest and smallest number.

Each time, we pop the largest number out and divide it by two then put it back to the priority queue, until the largest number becomes odd. We can not discard it and divide any other smaller numbers by two will only increase the max difference, so we can stop here.

ex1: [3, 5, 8] => [6, 8, 10] (pre-double) => [5, 6, 8] => [4, 5, 6] => [3, 4, 5] max diff is 5 – 3 = 2
ex2: [4,1,5,20,3] => [2, 4, 6, 10, 20] (pre-double) => [2, 4, 6, 10] => [2, 4, 5, 6] => [2,3,4,5] max diff = 5-2 = 3

Time complexity: O(n*logm*logn)
Space complexity: O(n)

C++/Set

class Solution {

public:

int minimumDeviation(vector<int>& nums) {

set<int> s;

for (int x : nums)

s.insert(x & 1 ? x * 2 : x);

int ans = *rbegin(s) - *begin(s);

while (*rbegin(s) % 2 == 0) {

s.insert(*rbegin(s) / 2);

s.erase(*rbegin(s));

ans = min(ans, *rbegin(s) - *begin(s));

}

return ans;

}

};

C++/PQ

class Solution {

public:

int minimumDeviation(vector<int>& nums) {

priority_queue<int> q;

int lo = INT_MAX;

for (int x : nums) {

x = x & 1 ? x * 2 : x;

q.push(x);

lo = min(lo, x);

}

int ans = q.top() - lo;

while (q.top() % 2 == 0) {

int x = q.top(); q.pop();

q.push(x / 2);

lo = min(lo, x / 2);

ans = min(ans, q.top() - lo);

}

return ans;

}

};

花花酱 LeetCode 1606. Find Servers That Handled Most Number of Requests

By zxi on October 3, 2020

You have k servers numbered from 0 to k-1 that are being used to handle multiple requests simultaneously. Each server has infinite computational capacity but cannot handle more than one request at a time. The requests are assigned to servers according to a specific algorithm:

The i^th (0-indexed) request arrives.
If all servers are busy, the request is dropped (not handled at all).
If the (i % k)^th server is available, assign the request to that server.
Otherwise, assign the request to the next available server (wrapping around the list of servers and starting from 0 if necessary). For example, if the i^th server is busy, try to assign the request to the (i+1)^th server, then the (i+2)^th server, and so on.

You are given a strictly increasing array arrival of positive integers, where arrival[i] represents the arrival time of the i^th request, and another array load, where load[i] represents the load of the i^th request (the time it takes to complete). Your goal is to find the busiest server(s). A server is considered busiest if it handled the most number of requests successfully among all the servers.

Return a list containing the IDs (0-indexed) of the busiest server(s). You may return the IDs in any order.

Example 1:

Input: k = 3, arrival = [1,2,3,4,5], load = [5,2,3,3,3] 
Output: [1] 
Explanation:
All of the servers start out available.
The first 3 requests are handled by the first 3 servers in order.
Request 3 comes in. Server 0 is busy, so it's assigned to the next available server, which is 1.
Request 4 comes in. It cannot be handled since all servers are busy, so it is dropped.
Servers 0 and 2 handled one request each, while server 1 handled two requests. Hence server 1 is the busiest server.

Example 2:

Input: k = 3, arrival = [1,2,3,4], load = [1,2,1,2]
Output: [0]
Explanation:
The first 3 requests are handled by first 3 servers.
Request 3 comes in. It is handled by server 0 since the server is available.
Server 0 handled two requests, while servers 1 and 2 handled one request each. Hence server 0 is the busiest server.

Example 3:

Input: k = 3, arrival = [1,2,3], load = [10,12,11]
Output: [0,1,2]
Explanation: Each server handles a single request, so they are all considered the busiest.

Example 4:

Input: k = 3, arrival = [1,2,3,4,8,9,10], load = [5,2,10,3,1,2,2]
Output: [1]

Example 5:

Input: k = 1, arrival = [1], load = [1]
Output: [0]

Constraints:

1 <= k <= 10⁵
1 <= arrival.length, load.length <= 10⁵
arrival.length == load.length
1 <= arrival[i], load[i] <= 10⁹
arrival is strictly increasing.

Solution: Heap + TreeSet

Use a min heap to store the release time -> server.
Use a treeset to track the current available servers.
For reach request, check whether servers can be released at that time.

Time complexity: O(nlogk)
Space complexity: O(k)

C++

// Author: Huahua

class Solution {

public:

vector<int> busiestServers(int k, vector<int>& arrival, vector<int>& load) {

priority_queue<pair<int, int>, vector<pair<int,int>>, greater<>> q; // {release_time, server}

set<int> servers;

vector<int> requests(k);

for (int i = 0; i < k; ++i)

servers.insert(i);

for (int i = 0; i < arrival.size(); ++i) {

const int t = arrival[i];

const int l = load[i];

// Release servers. O(logk) per pop()

while (!q.empty() && q.top().first <= t) {

servers.insert(q.top().second);

q.pop();

}

// Drop the request.

if (servers.empty()) continue;

// Find first avaiable one O(logk)

auto it = servers.lower_bound(i % k);

if (it == servers.end()) it = begin(servers);

const int idx = *it;

++requests[idx];

servers.erase(it);

q.emplace(t + l, idx);

}

const int max_req = *max_element(begin(requests), end(requests));

vector<int> ans;

for (int i = 0; i < k; ++i)

if (requests[i] == max_req) ans.push_back(i);

return ans;

}

};